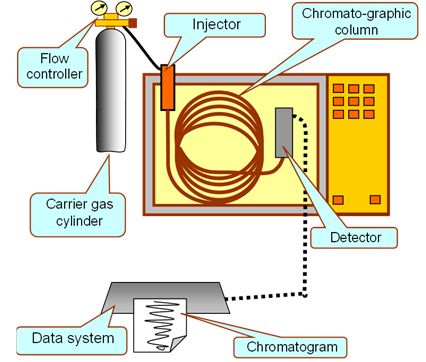
CONCEPTUALLY NEW MODEL OF GAS CHROMATOGRAPHY
DOI:
https://doi.org/10.15421/jchemtech.v32i3.308574Keywords:
adsorption, gas chromatography, van Deemter equation, concentration waves, separation of isotopesAbstract
The paper describes a mathematical model of gas chromatography in terms of a set concentration waves passing through an adsorption column. An analytical solution is derived for the passage of concentration eigenwaves through the entire adsorption column. This makes it possible to find analytical solutions for an arbitrarily shaped concentration signal passing through the adsorption column. To do this, it is necessary to decompose the input concentration signal into a set of adsorption column eigenwaves and to obtain an analytical solution for each of the concentration eigenwaves at the exit of that column. All of the concentration eigenwave solutions are then combined. This is the solution for passing an arbitrary concentration signal through the adsorption column. This approach is suitable for any periodic adsorption process and allows for the variable concentration of components at the entrance to the adsorption layer. The wave approach to the analysis of chromatographic column processes provides an explanation for the empirical Van Deemter equations used in the practice of gas chromatography.
References
Scott, R. P. W. (1998). Introduction to Analytical Gas Chromatography, 2nd ed. New York, USA: Marcel Dekker.
Jennings, W. G.; Mittlefehldt, E.; Stremple, P. (1997). Analytical Gas Chromatography, 2nd ed. New York, USA: Academic Press.
McNair, H. M. Miller, J. M. (1997). Basic Gas Chromatography. New York, USA: Wiley.
D. Rood. (1995). A practical guide to care, maintainance and troubleshooting of capillary gas chromatographic systems, 2nd edition. Heidelberg: Hüthig Verlag.
Harris W.E., Habgood H.W. (1967). Programmed Temperature Gas Chromatography, John Wiley, New York.
Modern Practice of Gas Chromatography, Fourth Edition. (2004). Edited by Robert L. Grob and Eugene F. Barry. New York, USA: John Wiley & Sons, Inc.
Kravchenko, M. B. (2023). Conceptualy new model of pressure swing adsorption. Journal of Chemistry and Technologies, 31(2), 353–367.
Kravchenko, M. B. (2014). Wave adsorption. Saarbruecken: Lap. Lambert Academic Publishing. (in Russian)
Ruthven, D.M. (1984). Principles of Adsorption and Adsorption Processes. New York: John Wiley & Sons.
Ruthven, D.M., Lee, L.-K., Yucel, H. (1980). Kinetics of non-isothermal sorption in molecular sieve crystals. AIChE J. 26, 6–23.
Ruthven, D. M., Farooq, S., Knaebel, K. S. (1994). Pressure Swing Adsorption. New York: VCH Publishers Inc.
Kravchenko, M. B. (2017). Wave mathematical model to describe gas chromatography. Refrigeration Engineering and Technology, 53(6), 28–37.
Kravchenko, M. B., Lavrenchenko, G. K. (2019). Effective Use of DP-PSA Gas Separation Technology. Chemical and Petroleum Engineering, 55(3-4), 298–305.
Kravchenko, M. B. (2018). Effect of Adsorbent Grain Size on the Pressure Swing Adsorption. Refrigeration Engineering and Technology, 54(1), 24–34.
Prigogine and R. Defay (1954). Chemical Thermodynamics, Chapter IV, translated by D.H. Everett, Longmans, Green & Co.
Mikusiński J. (1956). Operational calculus. Publishing. House of Foreign Literature, Moscow, 366p. (in Russian)
Bezverkhyy, I., Pujol, Q., Dirand, C., Herbst, F., Macaud, M. (2020). D2 and H2 adsorption capacity and selectivity in CHA zeolites: Effect of Si/Al ratio, cationic composition and temperature. Microporous and Mesoporous Materials, 302, 110217. ff10.1016/j.micromeso.2020.110217ff.ffhal-03006855ff
Kotoh, K., Nishikawa, T., Kashio, Y. (2002). Multi-component adsorption characteristics of hydrogen isotopes on synthetic zeolite 5A-type at 77.4K, J. Nuclear Sciance Technology. 39, 435–441.
Kotoh, K., Kimura, K., Nakamura, Y., Kudo, K. (2008). Hydrogen isotope separation using molecular sieve of synthetic zeolite 3A. Fusion Sciance Technology. 54, 419–422.
Giraudet, M., Bezverkhyy, I., Weber, G., Dirand, C., Macaud, M., Bellat, J.-P. (2018). D2/H2 adsorption selectivity on FAU zeolites at 77.4 K: Influence of Si/Al ratio and cationic composition. Microporous Mesoporous Mater. 270, 211–219.
Uda, T., Okuno, K., Suzuki, T., and Yuji. (1991). Naruse Gas chromatography for measurement of hydrogen isotopes at tritium processing. Journal of Chromatography, 586, 131–137.
Junboa, Z., Lipinga, G., Kuisheng, W. (2006). Hydrogen isotope separation by cryogenic gas chromatography using the combined column of 5A molecular sieve and Al2O3. International Journal of Hydrogen Energy, 31, 2131–2135.
Thakur, N., Aslani, S., Armstrong, D. W. (2021). Evaluation of gas chromatography for the separation of a broad range of isotopic compounds. Analytica Chimica Acta, 1165, 338490.
Kanao, E., Kubo, T., Naito, T., Sano, T., Yan, M., Tanaka, N., Otsuka, Tunable, K. (2020). Liquid chromatographic separation of H/D isotopologues enabled by aromatic p interactions, Anal. Chem. 92, 4065e4072.
Zhang, Y., Gao, B., Valdiviez, L., Zhu, C., Gallagher, T., Whiteson, K., Fiehn, O. (2021). Comparing Stable Isotope Enrichment by Gas Chromatography with Time-of-Flight, Quadrupole Time-of-Flight, and Quadrupole Mass Spectrometry. Anal. Chem., 93, 2174−2182.
Aerov M.E., Todes O.M. (1968). Hydraulic and thermal basics of devices with fixed and fluidized granular layer. Leningrad: Chemistry. (in Russian)
Vogd, R., Ringel, H., Hackfort, H., Schober T., Dieker, C. (1988). Gas Chromatographic Separation of Hydrogen Isotopes on Molecular Sieves. Fusion Technology, 14, 2P2A, 574–578. https://doi.org/10.13182/FST88-A25195
Downloads
Published
Issue
Section
License
Copyright (c) 2024 Oles Honchar Dnipro National University

This work is licensed under a Creative Commons Attribution 4.0 International License.
- Authors reserve the right of attribution for the submitted manuscript, while transferring to the Journal the right to publish the article under the Creative Commons Attribution License. This license allows free distribution of the published work under the condition of proper attribution of the original authors and the initial publication source (i.e. the Journal)
- Authors have the right to enter into separate agreements for additional non-exclusive distribution of the work in the form it was published in the Journal (such as publishing the article on the institutional website or as a part of a monograph), provided the original publication in this Journal is properly referenced
- The Journal allows and encourages online publication of the manuscripts (such as on personal web pages), even when such a manuscript is still under editorial consideration, since it allows for a productive scientific discussion and better citation dynamics (see The Effect of Open Access).